{%- extends "base_math.xhtml" -%}



{%- block title -%}Area Under the Curve of a Complex Integral{%- endblock -%}



{%- block footer -%}{{ math_footer ("integral.xhtml") }}{%- endblock -%}



{%- block style %}
    <link href="/css/integral.css" rel="stylesheet" />
{% endblock -%}



{%- block content %}
<h4>Area Under the Curve of a Complex Integral</h4>

<p>Scripts used to generate graphs: <a href="/dld/integral_scripts.zip">Link</a></p>

<h5>29/12/2018</h5>

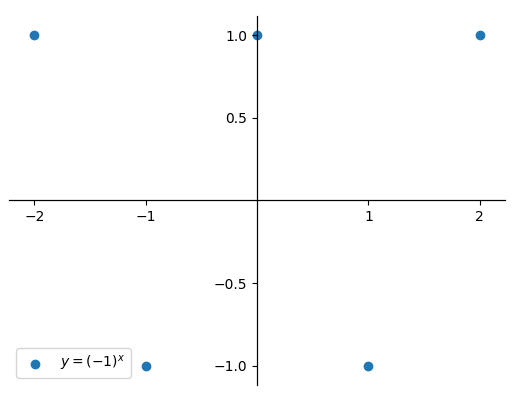
<p>A definite integral can be represented on the xy-plane as the signed area bounded by the curve of
the function f(x), the x-axis, and the limits of integration a and b. But it's not immediately clear
how this definition applies for complex valued functions.</p>

<p>Consider the following example:</p>

<div class="precontain"><div class="mathblock">
    <math xmlns="http://www.w3.org/1998/Math/MathML">
        <mrow>
            <munderover>
                <mo>&#x222b;</mo>
                <mn>0</mn>
                <mn>1</mn>
            </munderover>
            <msup>
                <mrow>
                    <mo>(</mo>
                    <mn>-1</mn>
                    <mo>)</mo>
                </mrow>
                <mi>x</mi>
            </msup>
            <mi>dx</mi>
        </mrow>
    </math>
</div></div>

<p>If the function is graphed on the xy-plane, the real valued outputs are sparse. Yet an elementary
antiderivative exists and the definite integral is well defined.</p>

<div class="figure">
    <img src="/img/minus_one_exp_x_real_values_only.png"
         alt="Real values only plot of minus one raised to the x power"
         height="400"
         width="520" />
    <div class="figcaption">Figure 1 - Real values only</div>
</div>

<p>In order to plot a meaningful graph that can be used to potentially calculate the integral as a
signed area, some cues are taken from Philip Lloyd's work on
<a href="https://phantomgraphs.weebly.com/" class="external">Phantom Graphs</a>. In that work, an
additional z-axis is used to extend the x-axis into a complex xz-plane, allowing complex inputs to
be graphed. For the function considered here, the z-axis is instead used to extend the y-axis into a
complex yz-plane to allow graphing of complex outputs instead.</p>

<p>Upon doing so, the following helical graph is obtained:</p>

<div class="figure">
    <img src="/img/minus_one_exp_x_full_plot.png"
         alt="Complete three dimensional graph of all values of minux one raised to the x power"
         height="400"
         width="520" />
    <div class="figcaption">Figure 2 - Full graph</div>
</div>

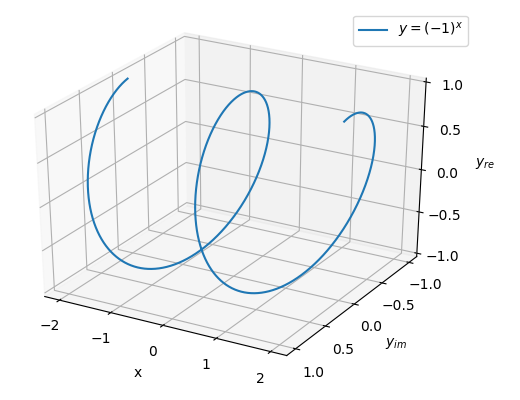
<p>The curve is continuous and spirals around the x-axis, intersecting with the real xy-plane at the
points plotted in the initial graph of Figure 1. However it is still not clear how to represent the
area under the curve.</p>

<p>Observing that complex numbers in cartesian form are composed of a real part and an imaginary
part, it is possible to decompose the function into real and imaginary components. These are easy to
obtain by rotating the graph above to view the real and imaginary parts as flat planes.</p>

<table id="component">
    <tr>
        <td>
            <div class="figure">
                <img src="/img/cos_pi_x.png"
                     alt="Graph of the real component of minus one raised to the power of x"
                     height="400"
                     width="520" />
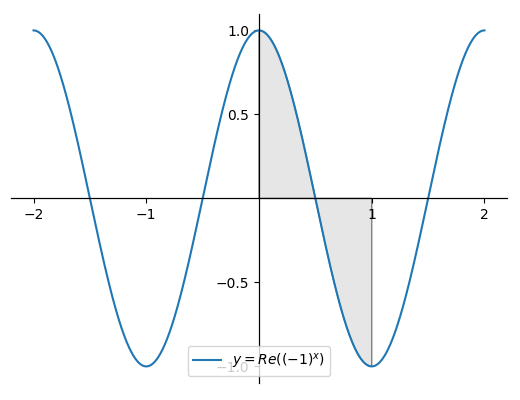
                <div class="figcaption">Figure 3 - Real component</div>
            </div>
        </td>
        <td>
            <div class="figure">
                <img src="/img/sin_pi_x.png"
                     alt="Graph of the imaginary component of minus one raised to the power of x"
                     height="400"
                     width="520" />
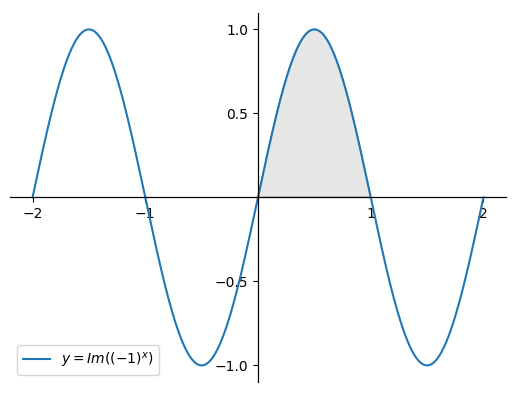
                <div class="figcaption">Figure 4 - Imaginary component</div>
            </div>
        </td>
    </tr>
</table>

<p>From this it can be seen that the function is a combination of a real valued cosine and an
imaginary valued sine. With the limits of integration under consideration, the real values disappear
and we are left with the following:</p>

<div class="precontain"><div class="mathblock">
    <table>
        <tr>
            <td colspan="2">
                <math xmlns="http://www.w3.org/1998/Math/MathML">
                    <mrow>
                        <munderover>
                            <mo>&#x222b;</mo>
                            <mn>0</mn>
                            <mn>1</mn>
                        </munderover>
                        <msup>
                            <mrow>
                                <mo>(</mo>
                                <mn>-1</mn>
                                <mo>)</mo>
                            </mrow>
                            <mi>x</mi>
                        </msup>
                        <mi>dx</mi>
                    </mrow>
                </math>
            </td>
        </tr>
        <tr>
            <td>=</td>
            <td>
                <math xmlns="http://www.w3.org/1998/Math/MathML">
                    <mrow>
                        <mi>i</mi>
                        <munderover>
                            <mo>&#x222b;</mo>
                            <mn>0</mn>
                            <mn>1</mn>
                        </munderover>
                        <mi>sin</mi>
                        <mrow>
                            <mo>(</mo>
                            <mi>&#x03c0;</mi>
                            <mo>&#x2062;</mo>
                            <mi>x</mi>
                            <mo>)</mo>
                        </mrow>
                        <mi>dx</mi>
                    </mrow>
                </math>
            </td>
        </tr>
        <tr>
            <td>=</td>
            <td>
                <math xmlns="http://www.w3.org/1998/Math/MathML">
                    <mrow>
                        <mo>-</mo>
                        <mfrac>
                            <mi>i</mi>
                            <mi>&#x03c0;</mi>
                        </mfrac>
                        <msubsup>
                            <menclose notation="right">
                                <mrow>
                                    <mi>cos</mi>
                                    <mrow>
                                        <mo>(</mo>
                                        <mi>&#x03c0;</mi>
                                        <mo>&#x2062;</mo>
                                        <mi>x</mi>
                                        <mo>)</mo>
                                    </mrow>
                                </mrow>
                            </menclose>
                            <mn>0</mn>
                            <mn>1</mn>
                        </msubsup>
                    </mrow>
                </math>
            </td>
        </tr>
        <tr>
            <td>=</td>
            <td>
                <math xmlns="http://www.w3.org/1998/Math/MathML">
                    <mrow>
                        <mo>-</mo>
                        <mfrac>
                            <mi>i</mi>
                            <mi>&#x03c0;</mi>
                        </mfrac>
                        <mrow>
                            <mo>(</mo>
                            <mn>-1</mn>
                            <mo>-</mo>
                            <mn>1</mn>
                            <mo>)</mo>
                        </mrow>
                    </mrow>
                </math>
            </td>
        </tr>
        <tr>
            <td>=</td>
            <td>
                <math xmlns="http://www.w3.org/1998/Math/MathML">
                    <mrow>
                        <mfrac>
                            <mrow>
                                <mn>2</mn>
                                <mi>i</mi>
                            </mrow>
                            <mi>&#x03c0;</mi>
                        </mfrac>
                    </mrow>
                </math>
            </td>
        </tr>
    </table>
</div></div>

<p>This agrees with the answer obtained by ordinary evaluation of the integral without considering
the graph, so the informal area under the curve definition still works. Considering the area under
the curve using polar coordinates also works, but requires evaluating a less than pleasant infinite
sum and so won't be considered here.</p>

<p>The next interesting question is how this relates to the surface area of a
<a href="https://www.mathcurve.com/surfaces.gb/helicoiddroit/helicoiddroit.shtml" class="external">
right helicoid</a>.</p>
{% endblock -%}